Answer:
The correct option is B.
Explanation:
Total possible digits are 0,1,2,3,4,5,6,7,8,9.
Total number of digits in a credit card = 16
It is given that that the last digit of credit card is fixed.
Total number of possible ways for 16th digit = 1
U-Kan-Trust-Us Credit Card numbers begin with 61, 62, or 63.
Total number of possible ways for first two digits = 3
Remaining places = 16 - 1 - 2 = 13
In these 13 remaining places any of 10 digits can be occur.
Total number of possible ways for remaining 13 digits =
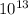
The maximum number of credit cards that UKTU can issue is
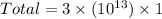
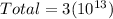
Therefore the correct option is B.