Question

a hockey puck has a mass of 1.0 kg. the coefficient of kinetic friction between the puck and the ice is 0.15 . a force of 2.6 n is applied horizontally to the puck to push it right. calculate the friction force

Answer 1

A hockey puck has a mass of 1.0 kg. The coefficient of kinetic friction between the puck and ice is 0.15. A force of 2.6 N is applied horizontally to the ...

Step-by-step explanation:

Answer 2

Approximately
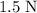`1.5\; {\rm N}`, assuming that the ice surface is level, the puck is moving, and that
`g = 9.8\; {\rm N \cdot kg^(-1)}`.

Step-by-step explanation:

With a mass of
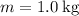`m = 1.0\; {\rm kg}`, the weight of this puck would be
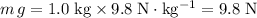`m\, g = 1.0\; {\rm kg} * 9.8\; {\rm N \cdot kg^(-1)} = 9.8\; {\rm N}` (downward in the vertical direction.)

The question stated that the push on the puck is horizontal. If the ice surface is level, the friction on the puck would also be horizontal. At the same time, the normal force on this puck would be vertical. The only forces on this puck in the vertical direction would be the normal force and the weight of the puck.

Since the puck is not moving vertically, the forces in the vertical direction (normal force and the weight of the puck) must be balanced. Thus, the normal force on this puck would be opposite to the weight of this puck. The magnitude of the normal force
`F_{\text{N}}` on this puck would be equal to the magnitude of the weight of the puck,
`9.8\; {\rm N}`.

The question states that the coefficient of kinetic friction is
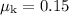`\mu_{\text{k}} = 0.15` between the ice and the puck. If the puck is moving, the magnitude of the friction between the ice and this puck would be
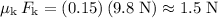`\mu_{\text{k}} \, F_{\text{k}} = (0.15)\, (9.8\; {\rm N}}) \approx 1.5\; {\rm N}`.