Question

A plane mirror and a concave mirror (f = 8.20 cm) are facing each other and are separated by a distance of 25.0 cm. An object is placed between the mirrors and is 12.5 cm from each mirror. Consider the light from the object that reflects first from the plane mirror and then from the concave mirror. What is the distance of the image (di) produced by the concave mirror?

Answer 1

Sol: - I1: Reflexión a la distancia del primer espejo del objeto = 5 cm a la derecha ==> distancia de la imagen = 5 cm a la izquierda Ahora, I2: Reflexión al espejo 2 distancia del objeto = 20-5 = 15 cm a la izquierda == > distancia de imagen = 15 cm a la derecha Ahora, I3: la imagen I2 será como objeto para el espejo 1 distancia de objeto = 15 + 20 = 35 cm a la derecha ==> distancia de imagen = 35 cm a la izquierda Ahora, I4: I1 la imagen será como objeto para el espejo 2 distancia del objeto = 5 + 20 = 25 cm a la izquierda ==> distancia de la imagen = 25 cm a la derecha Ahora, I5: la imagen I4 será como objeto para la distancia del espejo 1 ...

Step-by-step explanation:

Answer 2

The distance of image formed by the concave mirror is at 10.495 cm.

Step-by-step explanation:

Since we know that a plane mirror forms image at a distance behind the mirror at which the object is placed in front of the mirror thus we have.

Image formed by the plane mirror is at a distance of 12.5 cm behind the plane mirror.

The image formed by the plane mirror is at a distance of 12.5 + 25.0 = 37.5 cm from the pole of the concave mirror.

Thus by mirror formula we have

`(1)/(v)+(1)/(u)=(1)/(f)`

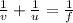
Using the standard sign convention we have

u = location of object = -37.5 cm

f = focal length of mirror = -8.20 cm

Thus using the given values we obtain

`(1)/(v)+(1)/(-37.5)=(1)/(-8.2)\\\\\therefore v=-10.495cm`

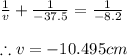
Thus the image is formed at a distance of 10.495 cm from the pole of mirror towards left of mirror.