Question

What is the value of 5^3i^9
-125i
-15i
15i
125i

Answer 1

`125i`

Explanation:

When we have potential expression that include imaginary numbers, we have to consider some basic results, because these imaginary potential expression are cyclical.

We know that:

`i=√(-1)`

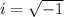
So, elevating both members to a third power, we have:

`i^(3)=(√(-1) )^(3)=\sqrt{(-1)^(3) }=√(-1)=i`

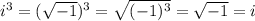
So,
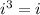`i^(3)=i`, which is the beginning, that's why we say that it's like a cycle.

So, from the problem, we have:

`5^(3) i^(9)`

To solve this, we consider the operations from the beginning:

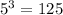`5^(3)=125`; and

`i^(9)=(i^(3))^(3)=(i)^(3)=i`; because
`i^(3)=i`

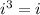
Therefore, the result would be
`125i`

Answer 2

Good morning ☕️

______

Answer:

125i

___________________

Explanation:

5³× i⁹ = 125 × i¹ = 125i

By the way :

i⁹ = (i⁴)²× i¹ = (1)²×i = i.

:)