Question

Find a basis for the vector space of all symmetric 3 x 3 matrices.

Answer 1

`\left[\begin{array}{ccc}0&1&0\\1&0&0\\0&0&0\end{array}\right],\left[\begin{array}{ccc}0&1&0\\1&0&0\\0&0&0\end{array}\right],\left[\begin{array}{ccc}0&1&0\\1&0&1\\0&1&0\end{array}\right]`

Explanation:

We are given that vector space of all symmetric matrix

M(R)={
`\left[\begin{array}{ccc}a&b&c\\d&e&f\\g&h&i\end{array}\right]`

where a,b,c,d,e,f,g,h,i
`\in R`}

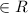Let A=
`\left[\begin{array}{ccc}a&b&c\\d&e&f\\g&h&i\end{array}\right]`

A'=
`\left[\begin{array}{ccc}a&d&g\\b&e&h\\c&f&i\end{array}\right]`

A is symmetric

Then A'=A

`\left[\begin{array}{ccc}a&b&c\\d&e&f\\g&h&i\end{array}\right]`=
`\left[\begin{array}{ccc}a&d&g\\b&e&h\\c&f&i\end{array}\right]`

a=a , e=e, i=i

a-a=0 , e-e=0, i-i=0

b=d, c=g ,f=h

Hence, the matrix

`\left[\begin{array}{ccc}0&b&c\\b&0&f\\c&f&0\end{array}\right]`

Therefore, the basis are

`\left[\begin{array}{ccc}0&1&0\\1&0&0\\0&0&0\end{array}\right],\left[\begin{array}{ccc}0&1&0\\1&0&0\\0&0&0\end{array}\right],\left[\begin{array}{ccc}0&1&0\\1&0&1\\0&1&0\end{array}\right]`

There are three elements to generate an element of vectors of all symmetric matrix
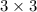`3* 3` matrix

`\left[\begin{array}{ccc}0&b&c\\b&0&f\\c&f&0\end{array}\right]`=
`b\left[\begin{array}{ccc}0&1&0\\1&0&0\\0&0&0\end{array}\right]+c\left[\begin{array}{ccc}0&1&0\\1&0&0\\0&0&0\end{array}\right]+f\left[\begin{array}{ccc}0&1&0\\1&0&1\\0&1&0\end{array}\right]`