Question

What is the energy released in this B- nuclear reaction 2K-> 2Ca0,e? (The atomic mass of 42 K is 41.962403 u and that of 42Ca is 41.958618 u)

Answer 1

Answer: The energy released in the given nuclear reaction is 3.526 MeV.

Step-by-step explanation:

For the given nuclear reaction:

`_(19)^(42)\textrm{K}\rightarrow _(20)^(42)\textrm{Ca}+_(-1)^(0)\textrm{e}`

We are given:

Mass of
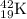`_(19)^(42)\textrm{K}` = 41.962403 u

Mass of
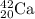`_(20)^(42)\textrm{Ca}` = 41.958618 u

To calculate the mass defect, we use the equation:

`\Delta m=\text{Mass of reactants}-\text{Mass of products}`

Putting values in above equation, we get:

`\Delta m=(41.962403-41.958618)=0.003785u`

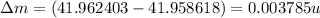
To calculate the energy released, we use the equation:

`E=\Delta mc^2\\E=(0.003785u)* c^2`

`E=(0.003785u)* (931.5MeV)` (Conversion factor:
`1u=931.5MeV/c^2` )

`E=3.526MeV`

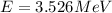
Hence, the energy released in the given nuclear reaction is 3.526 MeV.