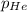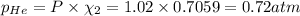Answer:
The total pressure of the mixture is 1.02 atm.
Partial pressure of the argon :
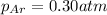
Partial pressure of the helium :
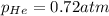
Step-by-step explanation:
Volume of argon in the bulb =
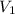 = 1.00 L
= 1.00 L
Pressure of argon in the bulb =
 = 0.75 atm
= 0.75 atm
Temperature of the both the gases = T
Moles of argon gases =
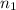
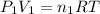 ..[1]
..[1]
Volume of helium in the bulb =
 = 1.50 L
= 1.50 L
Pressure of helium in the bulb =
 = 1.20 atm
= 1.20 atm
Moles of helium gases =
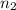
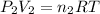 ..[2]
..[2]
[1] ÷ [2]
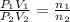
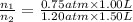
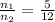
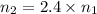
After opening the stopcock, the gases are mixed.
The mole fraction of argon =

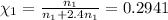
The mole fraction of helium=
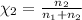
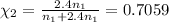
Volume of the mixture ,V= 1.00 L + 1.50 L =2.50 L
Total pressure in the mixture = P
Temperature is same = T
Total moles of gases in the mixtures = n

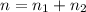
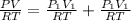
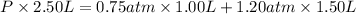
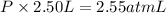
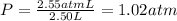
The total pressure of the mixture is 1.02 atm.
Partial pressure of the argon after mixing :
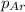
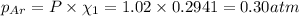
Partial pressure of the helium after mixing :