Question

A 40 lb box is suspended from two ropes which each make an angle of 45 degrees with the vertical. What is the tension (in pounds) on each rope?

Answer 1

The tension on each rope is 28.28 lb

Step-by-step explanation:

Given that,

Weight = 40 lb

Angle = 45°

We need to calculate the tension on each rope

Considering the symmetry of the system, the tension of each rope must be same

So,

`T\sin\theta+T\sin\theta=mg`

Put the value into the formula

`2T\sin\theta=mg`

`T*\sin45=(40)/(2)`

`T=(40√(2))/(2)`

`T=28.28\ lb`

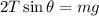
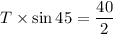
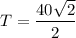
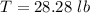
Hence, The tension on each rope is 28.28 lb.