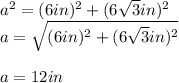Answer: The length of the hypotenuse is 12 inches.
Explanation:
You need to use the Pythagorean Theorem:
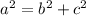
Where "a" is the hypotenuse and "b" and "c" are the legs of the right triangle.
You can say that:
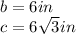
Therefore, substituting values into
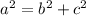 and solving for "a", we get that the lenght of the hypotenuse is:
and solving for "a", we get that the lenght of the hypotenuse is: