Question

A circle is inscribed in a regular hexagon with side length 10 feet. What is the area of the shaded region? Recall that in a 30 – 60 – 90 triangle, if the shortest leg measures x units, then the longer leg measures x units and the hypotenuse measures 2x units.

(150 – 75π) ft2
(300 – 75π) ft2
(150 – 25π) ft2
(300 – 25π) ft2

Answer 1

a

Explanation:

im god

Answer 2

The correct answer is the first option.

Explanation:

As the circle is inscribed in the hexagon their center points coincide. Now, draw lines from the center to the vertices of the hexagon, as the attached figure shows.

Notice that the 6 triangles are equals, because the hexagon is regular, and all are isosceles where the sides of the hexagons are their basis. Moreover, the angles that are opposite to the basis are all equals and their measure is 60 degrees. Thus, all triangles are equilateral.

With this facts we can calculate the area of the hexagon

`A_h = 6A_t`,

where
`A_t` stands for the area of one of the equilateral triangles. We know that an equilateral triangle with length of the side
`a`, its area is

`A_t = (√(3))/(4)a^2 .`

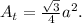
In this case
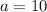`a=10` ft, thus

`A_t = (√(3))/(4)10^2 = 25√(3).`

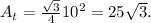
Then, the area of the hexagon is
`A_h = 6A_t = 150√(3)`.

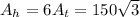Now, the height of one equilateral triangle is the radius of the circle, and it has length
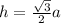`h = (√(3))/(2)a`, where
`a` stands for the length of the side of the equilateral triangle. Then,
`h = (√(3))/(2)10 =5√(3)`.

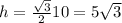The area of the circle is
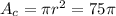`A_c = \pi r^2= 75\pi`.