Question

Orchard Farms has a pretax cost of debt of 7.29 percent and a cost of equity of 16.3 percent. The firm uses the subjective approach to determine project discount rates. Currently, the firm is considering a project to which it has assigned an adjustment factor of 1.25 percent. The firm's tax rate is 35 percent and its debt-equity ratio is .48. The project has an initial cost of $3.9 million and produces cash inflows of $1.26 million a year for 5 years. What is the net present value of the project?a. $421,619b. $446,556c. $514,370d. $561,027e. $478,721

Answer 1

Answer: Net present value = $446,556

Step-by-step explanation:

First we'll compute the Weighted Average Cost of Capital :

Weighted Average Cost of Capital =
`K_(e) * W_(e) + K_(d) * W_(d)`

= 0.163×
`(1)/(1.48)` + 0.0729× (1 - 0.35 )×
`(0.48)/(1.48)`

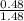
= 0.1255

where;

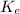`K_(e)` = Cost of equity

`W_(e)` = Proportion of equity

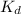`K_(d)` = Cost of debt

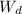`W_(d)` = Proportion of debt

Now, we'll compute the cost of capital using the following formula:

Cost of capital = Weighted Average Cost of Capital + adjustment factor

= 0.1255 + 0.0125

= 0.138 or 13.8%

∴ Net present value = Cash outflows - Total PV of cash flows

= $3,900,000 - $1,260,000 (Annuity value of 13.8% for 5 years)

`= 3,900,000 - 1260000 * ([1-(1+13.8)^(-5)])/(13.8)`

= $3,900,000 - $3,453,444

= $446,556

Therefore, the correct answer is option(b).