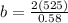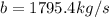Question

The suspension system of a 2100 kg automobile "sags" 8.5 cm when the chassis is placed on it. Also, the oscillation amplitude decreases by 63% each cycle. Estimate the values of (a) the spring constant k and (b) the damping constant b for the spring and shock absorber system of one wheel, assuming each wheel supports 525 kg.

Answer 1

Part a)

`k = 6.06 * 10^4 N/m`

Part b)

`b = 1795.4 kg/s`

Step-by-step explanation:

Part a)

as the mass of the suspension system is given as

`m = 2100 kg`

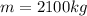
also we have

`x = 8.5 cm`

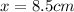
so now for force balance we have

`mg = kx`

`(525)(9.81) = k(0.085)`

`k = 6.06 * 10^4 N/m`

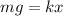
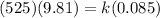
Part b)

Now we know that amplitude decreases by 63% in each cycle

so after one cycle the amplitude will become 37% of initial amplitude

so it is given as

`A = 0.37 A_o`

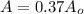
also we know

`A = A_o e^(-bt/2m)`

`0.37 A_o = A_o e^(-bt/2m)`

`(bt)/(2m) = 1`

`b = (2m)/(t)`

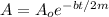
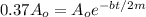
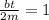
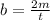
here t = time period of one oscillation

so it is

`t = 2\pi\sqrt{(m)/(k)}`

`t = 2\pi\sqrt{(525)/(6.06 * 10^4)}`

`t = 0.58 s`

now damping constant is

`b = (2(525))/(0.58)`

`b = 1795.4 kg/s`