Step-by-step explanation:
Potential difference, V = 1878.7197 volts
Energy gained by the electron when it is accelerating is, eV
Let v is the velocity and m is the mass of the electron. So,
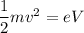

e is the charge on electron
m is the mass of electron
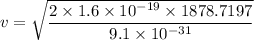

Speed of light,

Required percentage of the speed of light,

So, the electron be moving after being accelerated through a potential difference of 1,878.7197 volts is 8.56 % of the speed of light.