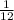Answer:
The probability that you will get a sum of 4 before you get a sum of 5 is
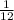
Explanation:
Given : If you roll two fair dice repeatedly.
To find : What is the probability that you will get a sum of 4 before you get a sum of 5 ?
Solution :
When two dice are rolled the outcomes are
(1,1) (1,2) (1,3) (1,4) (1,5) (1,6)
(2,1) (2,2) (2,3) (2,4) (2,5) (2,6)
(3,1) (3,2) (3,3) (3,4) (3,5) (3,6)
(4,1) (4,2) (4,3) (4,4) (4,5) (4,6)
(5,1) (5,2) (5,3) (5,4) (5,5) (5,6)
(6,1) (6,2) (6,3) (6,4) (6,5) (6,6)
Total number of outcomes = 36
Favorable outcome get a sum of 4 before you get a sum of 5 is (1,3) ,(2,2) and (3,1) = 3
The probability that you will get a sum of 4 before you get a sum of 5 is
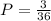
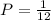
Therefore, The probability that you will get a sum of 4 before you get a sum of 5 is