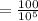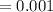Question

Six digits are chosen randomly from the set {0,1,2,…,9} a.) What is the probability that all six digits are the same? b.) What is the probability that five or more of the digits of the same?

Answer 1

a) 0.00001

b) 0.001

Explanation:

Given numbers,

0, 1, 2, 3, 4, 5, 6, 7, 8, 9

a) If by these numbers we have to make six digit number,

So, the total ways of arranging 6 digit number =
`10^6`

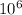
Now, if all six digit in the number are same,

Then the possible ways = 10 ( eg : 000000, 111111, 222222, 333333, 444444... etc ),

Hence, the probability that all six digits are the same

`=(10)/(10^6)=(1)/(10^5)=0.00001`

b) The ways that five or more of the digits of the same = exactly 5 digits are same + Exactly 6 digits are same

∵ The ways that 5 digits are same = 10 × 9 = 90 (i.e. 111110, 111112, 111113,.....9 times, 222220, 222221,.....9 times .... etc..)

So, the number of ways that 5 or more than 5 digits are same = 90 + 10 = 100

Hence, the probability that five or more of the digits of the same

`=(100)/(10^5)`

`=0.001`