Answer:
2.4 watt
Step-by-step explanation:
Case 1 :
n = Number of bulbs = 60
V = Voltage of the source = 240 volts
P = Power dissipated by the bulbs = 2 W
R = Resistance of each bulb
R' = Resistance of the combination of the bulbs = 60 R
Power dissipated is given as
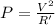
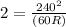
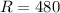 ohm
ohm
After 10 bulbs are removed
Case 2 :
n' = Number of bulbs in series = 50
V = Voltage of the source = 240 volts
P = Power dissipated by the bulbs = ?
R = Resistance of each bulb = 480 ohm
R' = Resistance of the combination of the bulbs = 50 R = (50) (480) = 24000 ohm
Power dissipated is given as
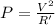
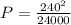
 Watt
Watt