Answer:
Yes,
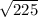 is a rational number.
is a rational number.
Explanation:
We are asked to determine whether the square root of 225 is a rational number or not.
We know that a number is a rational, when it can be written as a fraction.
Let us find square root of 225.
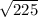
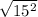
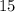
Since 15 can be written as
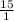 , therefore,
, therefore,
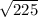 is a rational number.
is a rational number.