Question

Find the total binding energy of the mass C = 12.000000 u. nucleus (Z =6), given the atomic A. 127.6 MeV B. 104.6 MeV C. 931.5 MeV D. 92.2 MeV E. 89.1 MeV

Answer 1

Answer: The correct answer is Option D.

Step-by-step explanation:

We are given a nucleus having representation:
`_6^(12)\textrm{C}`

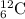
Number of protons = 6

Number of neutrons = 12 - 6 = 6

Number of electrons = 6

To calculate the mass defect of the nucleus, we use the equation:

`\Delta m=[(n_p* m_p)+(n_n* m_n)+(n_e* m_e)]-M`

where,

`n_p` = number of protons = 6

`m_p` = mass of one proton = 1.00728 u

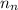`n_n` = number of neutrons = 6

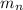`m_n` = mass of one neutron = 1.00866 u

`n_e` = number of electrons = 6

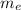`m_e` = mass of one electron = 0.00054858 u

M = Mass number = 12

Putting values in above equation, we get:

`\Delta m=[(6* 1.00728)+(6* 1.00866)+(6* 0.00054858)]-12\\\\\Delta m=0.098931u`

To calculate the binding energy of the nucleus, we use the equation:

`E=\Delta mc^2\\E=(0.098931u)* c^2`

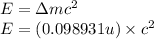
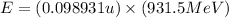`E=(0.098931u)* (931.5MeV)` (Conversion factor:
`1u=931.5MeV/c^2` )

`E=92.2MeV`

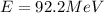
Hence, the correct answer is Option D.