Question

A steel sphere (SG = 7.8) of 13 mm diameter falls at a constant velocity of 0.6 m/s through an oil (SG = 0.90). Calculate the viscosity of the oil assuming that the fall occurs in a large tank and that the Reynolds number is very small.

Answer 1

1.058 decapoise

Step-by-step explanation:

The specific gravity of a substance is equal to the density of substance in CGS system and if the specific gravity is multiplied by 1000 then we get the density of substance in MKS system.

Density of steel, ρ = 7800 kg/m^3

density of oil, σ = 900 kg/m^3

v = 0.6 m/s

diameter = 13 mm

radius, r = 6.5 mm

Let η be the coefficient of viscosity.

Use the formula for the terminal velocity

`v=(2r^(2)(\rho -\sigma )g)/(9\eta)`

`\eta =(2r^(2)(\rho -\sigma )g)/(9v)`

`\eta =(2*6.5*10^(-3)*6.5*10^(-3)(7800-900)*9.8)/(9*0.6)`

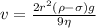
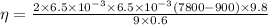
η = 1.058 decapoise