Question

A personal computer manufacturer buys 36% of its chips from Japan and the rest from the United States. Of the Japanese chips, 1.9% are defective, and 1.2% of the American chips are defective.

Find the probability that a chip is defective. (Round your answer to four decimal places.)?

Answer 1

The probability is 0.0145.

Explanation:

We first calculate two probabilities:

• the probability that a faulty chip is coming from Japan, denoted by P(D∪J), and
• the probability that a faulty chip is coming from the US, denoted by P(D∪A).

And then we sum them up.

Given that the probability of finding a defective chip is conditioned by the probabilities of the chips come from, we deduce that we'll have to use the formulae for extraction without replacement:

P(D∪J) = P(J)*P(D/J)

P(D∪A) = P(A)*P(D/A)

We know that

`P(J) = 0.36\\ P(A)=0.64\\ P(D/J)=0.019\\ P(D/A)=0.012`

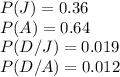
So we can simply calculate

P(D∪J)+P(D∪A) =
`P(J)*P(D/J)+P(A)*P(D/A)`

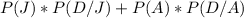
P(D∪J)+P(D∪A) =
`0.36*0.019+0.64*0.012 = 0.01452`

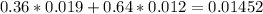
Therefore the rounded answer to 4 decimals would be 0.0145.