Question

A 1 mW laser beam is incident onto a detector. Determine the fractional fluctuation in number of photons intercepted by the detector in a time interval T 1 us for photons with energy (a) E1 10 Me; (b) Ez 10 eV.

Answer 1

(a)
`625 photons`

(b)
`625* 10^(6)photons`

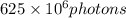
Step-by-step explanation:

Given that , the power of the laser beam is,

`P=1 mW\\P=1* 10^(-3) W`

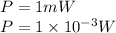
and time is given is,

`t=1\mu s\\t=10^(-6)s`

Now the energy formula for the laser beam is,

`E=P* t`

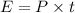
Now,

`E=10^(-3)* 10^(-6)  \\E=10^(-9)J`

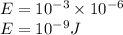
(a) The value of energy is given,

`E_(1)=10MeV\\E_(1)=10* 10^(6)* 1.6* 10^(-19)J\\ E_(1)=16* 10^(-13)J`

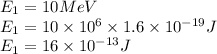
Now the no of photons's fraction fluctuation is,

`n=(E)/(E_(1) )\\ n=(10^(-9) )/(16* 10^(-13) )\\ E_(1)=625 photons`

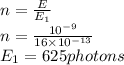
Therefore the no of photons is
`625 photons`.

(b)The value of energy is given,

`E_(z)=10eV\\E_(z)=16* 10^(-19)J`

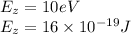
Now the no of photons's fraction fluctuation is,

`n=(E)/(E_(z) )\\ n=(10^(-9) )/(16* 10^(-19) )\\n=625* 10^(6)photons`

Therefore the no of photons is
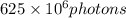`625* 10^(6)photons`.