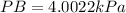Answer:
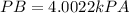
Step-by-step explanation:
For an ideal fluid (water in this case) we can use the Bernoulli equation to solve this exercise.
For two different points A and B inside an ideal fluid we can write that :
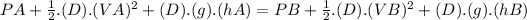
Where P is the pressure in that point.
Where D is the density of the fluid.
Where V is the speed of the fluid at that point.
Where g is the acceleration due to gravity.
Where h is the hight respect to a comparison plane.
The Bernoulli equation is an energy equation.
PA represents the energy due to pressure in the point A.
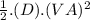 represents the kinetic energy in the point A.
represents the kinetic energy in the point A.
And
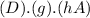 represents the potential energy in the point A.
represents the potential energy in the point A.
We are going to use another equation. Given that we are considering the water as an ideal fluid, the flow is constant in the pipe.
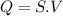
Q is the flow.
S is the section of the pipe and V is the speed of the fluid
Given that is constant, between two points A and B inside the pipe :
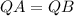 ⇒
⇒
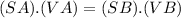 (I)
(I)
The section of the pipe given its diameter can be calculated as :

Where d is the diameter in that section.
Now, we write the Bernoulli equation between the two points. Given that this is a horizontal pipe, the potential energy term will be canceled because the two points will have the same potential energy respect to a comparison plane.
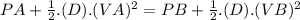 (II)
(II)
If we use (I) :
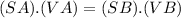 ⇒
⇒
 ⇒
⇒
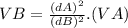 (III)
(III)
Using (III) in (II) :


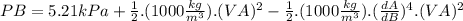
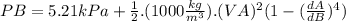
Replacing the values of the diameters dA and dB we obtain that the pressure in B is