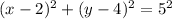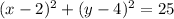Answer:
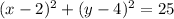
Explanation:
step 1
Find the diameter of the circle
the formula to calculate the distance between two points is equal to
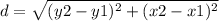
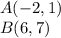
substitute the values
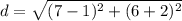
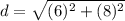
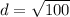
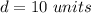
The radius is half the diameter
so
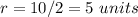
step 2
Find the center of the circle
the center of the circle is the midpoint between the endpoints of the diameter
so
The center is

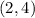
step 3
Find the equation of the circle
The equation of the circle is
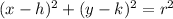
substitute the values