Question

Sunlight strikes a piece of crown glass at an angle of incidence of 30.9°. Calculate the difference in the angle of refraction between a red (660 nm) and a blue (470 nm) ray within the glass. The index of refraction is n=1.520 for red and n=1.531 for blue light. The ray now travels inside the glass. What is the minimum angle of incidence at which the red ray can hit the surface of the glass and become there totally internally reflected and not refracted?

Answer 1

Difference in the angle of refraction = 0.5163°

41.1399 is the minimum angle of incidence.

Step-by-step explanation:

Angle of incidence = 30.9°

For blue light :

Using Snell's law as:

`\frac {sin\theta_2}{sin\theta_1}=\frac {n_1}{n_2}`

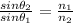
Where,

Θ₁ is the angle of incidence

Θ₂ is the angle of refraction

n₁ is the refractive index for blue light which is 1.531

n₂ is the refractive index of air which is 1

So,

`\frac {sin\theta_2}{sin{30.9}^0}=\frac {1.531}{1}`

`{sin\theta_2}=0.7862`

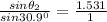
Angle of refraction for blue light = sin⁻¹ 0.7862 = 51.8318°.

For red light :

Using Snell's law as:

`\frac {sin\theta_2}{sin\theta_1}=\frac {n_1}{n_2}`

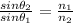
Where,

Θ₁ is the angle of incidence

Θ₂ is the angle of refraction

n₁ is the refractive index for red light which is 1.520

n₂ is the refractive index of air which is 1

So,

`\frac {sin\theta_2}{sin{30.9}^0}=\frac {1.520}{1}`

`{sin\theta_2}=0.7806`

Angle of refraction for red light = sin⁻¹ 0.7806 = 51.3155°.

The difference in the angle of refraction = 51.8318° - 51.3155° = 0.5163°

Calculation of the critical angle for the red light for the total internal reflection to occur :

The formula for the critical angle is:

`{sin\theta_(critical)}=\frac {n_r}{n_i}`

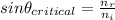
Where,

`{\theta_(critical)}` is the critical angle

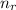`n_r` is the refractive index of the refractive medium.

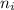`n_i` is the refractive index of the incident medium.

n₁ is the refractive index for red light which is 1.520 (incident medium)

n₂ is the refractive index of air which is 1 (refractive medium)

Applying in the formula as:

`{sin\theta_(critical)}=\frac {1}{1.520}`

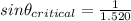
The critical angle is = sin⁻¹ 0.6579 = 41.1399°