Question

(Prob. 5.32) The industrial engineering department for Invade Air Fresheners has found that a new packing machine should save $45,000 for each of the next 8 years. The machine will require a major overhaul at the end of 5 years costing $12,000. The machine’s expected salvage value after 8 years is 7.5% of its original cost. If money is worth 5%, determine the amount Invade should be willing to pay for the machine.

Answer 1

It will be willing to pay up to $297,853.46

Step-by-step explanation:

First, we calculate present value of the cash saving

`C * (1-(1+r)^(-time) )/(rate) = PV\\`

C 45000

time 8

rate 0.05

`45000 * (1-(1+0.05)^(-8) )/(0.05) = PV\\`

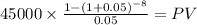
PV $290,844.57

Then, the present Value of the salvage value

`(Salvage)/((1 + rate)^(time) ) = PV`

Maturity 7.50 %

time 8.00

rate 0.05

`(7.5)/((1 + 0.05)^(8) ) = PV`

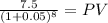
PV 5.08 %

This is calculate as a percent, because we are not given with a cash value.

Last, the 12,000 major overhaul

`(Overhaul)/((1 + rate)^(time) ) = PV`

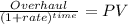
Maturity -12,000.00

time 8.00

rate 0.05

`(12000)/((1 + 0.05)^(8) ) = PV`

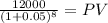
PV -8,122.07

This PV is negative as it is a cash out-flow

Lastly, we add them all:

290,844.57 + 0.0508PV - 8,122.07 = PV

And solve for PV

290,844.57 - 8,122.07 = PV - 0.0508PV

282,722.5‬ = 0.9492PV

282,722.5/0.9492 = PV

PV = 297,853.455541 = 297,853.46