Answer:
Minimum diameter of the camera lens is 22.4 cm
The focal length of the camera's lens is 300cm
Step-by-step explanation:
y = Resolve distance = 0.3 m
h = Height of satellite = 100 km
λ = Wavelength = 550 nm
Angular resolution
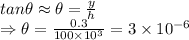
From Rayleigh criteria

Minimum diameter of the camera lens is 22.4 cm
Relation between resolvable feature, focal length and angular resolution
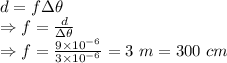
The focal length of the camera's lens is 300cm