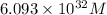Question

An electrochemical cell at 25°C is composed of pure copper and pure lead solutions immersed in their respective ionis. For a 0.6 M concentration of Cu2+, the lead electrode is oxidized yielding potential of 0.507 V. a cell a) Calculate the concentration of Pb2+ b) Suppose the lead electrode is reduced, in that case what would be the concentration of Pb2 What does this answer tell you?

Answer 1

(a) The concentration of
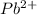`Pb^(2+)` is, 0.0337 M

(b) The concentration of
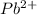`Pb^(2+)` is,
`6.093* 10^(32)M`

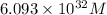
Solution :

(a) As per question, lead is oxidized and copper is reduced.

The oxidation-reduction half cell reaction will be,

Oxidation half reaction:
`Pb\rightarrow Pb^(2+)+2e^-`

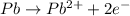
Reduction half reaction:
`Cu^(2+)+2e^-\rightarrow Cu`

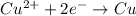
The balanced cell reaction will be,

`Pb(s)+Cu^(2+)(aq)\rightarrow Pb^(2+)(aq)+Cu(s)`

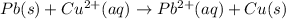
Here lead (Pb) undergoes oxidation by loss of electrons, thus act as anode. Copper (Cu) undergoes reduction by gain of electrons and thus act as cathode.

First we have to calculate the standard electrode potential of the cell.

`E^o_([Pb^(2+)/Pb])=-0.13V`

`E^o_([Cu^(2+)/Cu])=+0.34V`

`E^o=E^o_([Cu^(2+)/Cu])-E^o_([Pb^(2+)/Pb])`

`E^o=0.34V-(-0.13V)=0.47V`

Now we have to calculate the concentration of
`Pb^(2+)`.

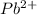Using Nernest equation :

`E_(cell)=E^o_(cell)-(0.0592)/(n)\log ([Pb^(2+)])/([Cu^(2+)])`

where,

n = number of electrons in oxidation-reduction reaction = 2

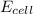`E_(cell)` = 0.507 V

Now put all the given values in the above equation, we get:

`0.507=0.47-(0.0592)/(2)\log ([Pb^(2+)])/((0.6))`

`[Pb^(2+)]=0.0337M`

Therefore, the concentration of
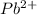`Pb^(2+)` is, 0.0337 M

(b) As per question, lead is reduced and copper is oxidized.

The oxidation-reduction half cell reaction will be,

Oxidation half reaction:
`Cu\rightarrow Cu^(2+)+2e^-`

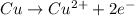
Reduction half reaction:
`Pb^(2+)+2e^-\rightarrow Pb`

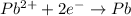
The balanced cell reaction will be,

`Cu(s)+Pb^(2+)(aq)\rightarrow Cu^(2+)(aq)+Pb(s)`

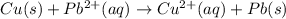
Here Copper (Cu) undergoes oxidation by loss of electrons, thus act as anode. Lead (Pb) undergoes reduction by gain of electrons and thus act as cathode.

First we have to calculate the standard electrode potential of the cell.

`E^o_([Pb^(2+)/Pb])=-0.13V`

`E^o_([Cu^(2+)/Cu])=+0.34V`

`E^o=E^o_([Pb^(2+)/Pb])-E^o_([Cu^(2+)/Cu])`

`E^o=-0.13V-(0.34V)=-0.47V`

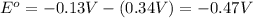
Now we have to calculate the concentration of
`Pb^(2+)`.

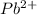Using Nernest equation :

`E_(cell)=E^o_(cell)-(0.0592)/(n)\log ([Cu^(2+)])/([Pb^(2+)])`

where,

n = number of electrons in oxidation-reduction reaction = 2

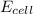`E_(cell)` = 0.507 V

Now put all the given values in the above equation, we get:

`0.507=-0.47-(0.0592)/(2)\log ((0.6))/([Pb^(2+)])`

`[Pb^(2+)]=6.093* 10^(32)M`

Therefore, the concentration of
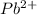`Pb^(2+)` is,
`6.093* 10^(32)M`