Question

Lead atoms occupy a volume of 3 x 10-29 m3. Each atom contributes two free electrons. Calculate the Fermi velocity of lead.

Answer 1

Answer: The Fermi velocity of lead is 64.4 km/s.

Step-by-step explanation:

To calculate the Fermi velocity, we use the equation:

`V_f=(h)/(2\pi m_e)((3\pi^2N)/(V))^(1/3)`

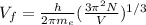
where,

h = Planck's constant =
`6.62* 10^(-34)Js`

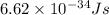
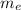`m_e` = mass of electron =
`9.1* 10^(-31)kg`

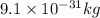
N = Number of atoms present in per volume of atom multiplied by number of electrons present in given atom =
`(2* N_A* V)/(M)`

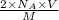
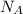`N_A` = Avogadro's number =
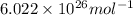`6.022* 10^(26)mol^(-1)` (When the mass is in kilograms)

V = Volume =
`3* 10^(-29)m^3`

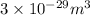
M = molecular weight of lead = 207.2 g/mol

Putting values in above equation, we get:

`V_f=(6.62* 10^(-34))/(2* 3.14* (9.1* 10^(-31)))((3* (3.14)^2* (2* 6.022* 10^(23)* 3* 10^(-29)))/(3* 10^(-29)* 207.2))^(1/3)`

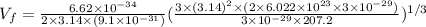
`V_f=0.0644* 10^6m/s=64.4km/s` (Conversion factor: 1 km = 1000 m)

Hence, the Fermi velocity of lead is 64.4 km/s