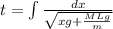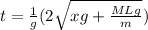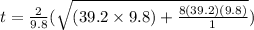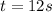Answer:
Part a)

Part b)
t = 12 s
Step-by-step explanation:
Part a)
Tension in the rope at a distance x from the lower end is given as
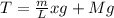
so the speed of the wave at that position is given as

here we know that

now we have
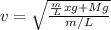

Part b)
time taken by the wave to reach the top is given as