Question

Television sizes are usually described by the length of their diagonal measure. What would be the listed size of the TV shown in the picture?

A. 1,424 inches

B. 32 inches

C. 38 inches

D. 20 inches

Answer 1

Let D = diagonal distance of tv.

D^2 = (32)^2 + (20)^2

D^2 = 1424

sqrt{D^2} = sqrt{1424}

D = 37.7359245282

We can round off to the nearest ones place.

D = 38 inches is the answer.

Answer 2

C. 38 inches.

Explanation:

We have been given that television sizes are usually described by the length of their diagonal measure. We are asked to find the listed size of the TV shown in the picture.

We can see that the length of screen is 32 inches and width is 20 inches. To find the listed size of the TV, we will use Pythagoras theorem as:

`\text{Diagonal}^2=32^2+20^2`

`\text{Diagonal}^2=1024+400`

`\text{Diagonal}^2=1424`

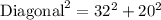
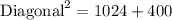
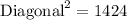
Take square root of both sides:

`\text{Diagonal}=√(1424)`

`\text{Diagonal}=37.7359245282`

`\text{Diagonal}\approx 38`

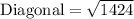
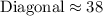
Therefore, the listed size of the TV would be 38 inches and option C is the correct choice.