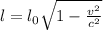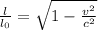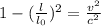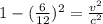Question

When at rest, a ruler has a length of 12.0 inches. How fast (in m/s) must the ruler fly past you in order for it to shorten to 6.00 inches in length? Hint: to use scientific notation within Canvas, use e+ instead of 10^ (ex. 3.00×108 = 3.00e+8).

Answer 1

2.598×
`10^8 m/sec`

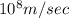
Step-by-step explanation:

The length of the contraction is given by
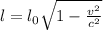`l=l_0\sqrt{1-(v^2)/(c^2)}` here
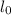`l_0` is length of the ruler at rest which is given as 12 inches

l is the length of the ruler in moving condition which is given as 6 inches c is the speed of the light and v is the velocity of the ruler.

`l=l_0\sqrt{1-(v^2)/(c^2)}`

`(l)/(l_0)=\sqrt{1-(v^2)/(c^2)}`

`1-((l)/(l_0))^2=(v^2)/(c^2)`

`1-((6)/(12))^2=(v^2)/(c^2)`

`0.866=(v)/(c)`

`v=0.866* 3* 10^8=2.598* 10^8 m/sec`