Question

A force of 8800 N is exerted on a piston that has an area of 0.010 m^2. What is the force exerted by a second piston that has an area of 0.040 m^2?

Answer 1

the force exerted by a second piston is 35200 N

Step-by-step explanation:

Answer 2

Step-by-step explanation:

It is given that,

Force on piston, F₁ = 8800 N

Area,
`A_1=0.01\ m^2`

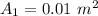
Area,
`A_2=0.04\ m^2`

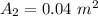
Let F₂ is the force exerted on the second piston. Using Pascal's law as :

Pressure at piston 1 = Pressure at piston 2

`(F_1)/(A_1)=(F_2)/(A_2)`

`F_2=(F_1)/(A_1)* A_2`

`F_2=(8800)/(0.01)* 0.04`

`F_2=35200\ N`

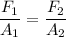
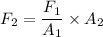
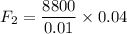
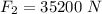
So, the force exerted by a second piston is 35200 N. Hence, this is the required solution.