Answer:
D= R
R =(6,∞)
A: y=6
Explanation:
We are asked to Find the domain (D ) range(R) and Asymptote (A) of the graph
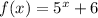
Our function
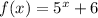 is an translated function of
is an translated function of
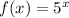 which is an exponential function. Therefore the basic properties remains the same.
which is an exponential function. Therefore the basic properties remains the same.
Any exponential function is defined for all values of x and Hence
c
however, The range of exponential functions is always positive from 0 to infinity . however here we have 6 added to out parent exponential function and hence the Range of exponential function is always be
Range = (6,∞)
Asymptote : Asymptote of our parent exponential function is y = 0, but in our function
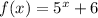 , the asymptote will be y=6
, the asymptote will be y=6