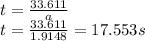Answer:
A) The minimum constant acceleration is
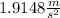
B) The airplane takes 17.553 seconds to become airborne.
Step-by-step explanation:
First, consider the basic equations for the motion with constant acceleration:

Where
 and
and
 are the initial position and velocity respectively; a is the acceleration and t the time.
are the initial position and velocity respectively; a is the acceleration and t the time.
Let's imagine that the aircraft had become airborne after the 295 m run, and let's model this movement by the last equations. The airplane is considered to be at position zero (
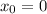 and start from repose (it's start velocity is zero:
and start from repose (it's start velocity is zero:
 )
)
Let's convert the velocity to m/s:
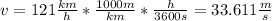
The airplane has a velocity of 33.611 m/s and has run 295 meters when it becomes airborne, so at that time:
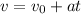
 (The initial velocity is zero)
(The initial velocity is zero)
 (The initial position is zero)
(The initial position is zero)
At this point we have a two-variable-two-equation system; if we could solve it we would know the time and the acceleration.
Let's isolate the time from the first equation and replace it in the second one.

Then, find the acceleration:

Now, it is possible to find the time by means of any of the initial equations we wrote. Let's take, for example, the first one: