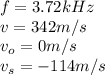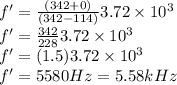Question

An airplane traveling at one third the speed of sound (i.e., 114 m/s) emits a sound of frequency 3.72 kHz. At what frequency does a stationary listener hear the sound as the plane approaches? Answer in units of kHz.

Answer 1

f'=5.58kHz

Step-by-step explanation:

This is an example of the Doppler effect, the formula is:

`f'=((v+v_o))/((v+v_s))f`

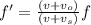
Where f is the actual frequency,
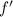`f'` is the observed frequency,
`v` is the velocity of the sound waves,
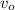`v_o` the velocity of the observer (which is negative if the observer is moving away from the source) and
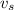`v_s` the velocity of the source (which is negative if is moving towards the observer). For this problem:

`f=3.72kHz\\v=342m/s\\v_o=0m/s\\v_s=-114m/s`

`f'=((342+0))/((342-114))3.72*10^3\\f'=(342)/(228)3.72*10^3\\f'=(1.5)3.72*10^3\\f'=5580Hz=5.58kHz`