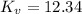Question

Take water density and kinematic viscosity as p=1000 kg/m3 and v= 1x10^-6 m^2/s. (c) Water flows through an orifice plate with a loss coefficient, Ko, of 10 and diameter of Do= 45 mm. The flow is controlled by a gate valve downstream of the plate with a pipe diameter Dy 1.5Do. Assuming that the total head drop across both components is Δhtotal=25 m, determine the loss coefficient of the valve to maintain a flow rate through the system of 10 I/s. Ignore the friction loss.

Answer 1

`K_v=12.34`

Step-by-step explanation:

Given;

For orifice, loss coefficient, K₀ = 10

Diameter, D₀ = 45 mm = 0.045 m

loss coefficient of the orifice, Ko = 10

Diameter of the gate valve, Dy = 1.5D₀ = 1.5 × 0.045 m = 0.0675 m

Total head drop, Δhtotal=25 m

Discharge, Q = 10 l/s = 0.01 m³/s

Now,

the velocity of flow through orifice, Vo = Discharge / area of the orifice

or

Vo =
`(0.01)/((\pi)/(4)0.045^2)`

or

Vo = 6.28 m/s

also,

the velocity of flow through gate valve,
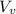`V_v` = Discharge / area of the orifice

or

`V_v` =
`(0.01)/((\pi)/(4)0.0675^2)`

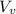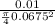
or

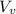`V_v` = 2.79 m/s

Now,

the total head drop = head drop at orifice + head drop at gate valve

or

25 m =
`K_o(V_o^2)/(2g)+K_v(V_v^2)/(2g)`

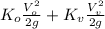
where,

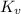`K_v` is the loss coefficient for the gate valve

on substituting the values, we get

25 m =
`10(6.28^2)/(2* 9.81)+K_v(2.79^2)/(2*9.81)`

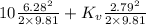
or

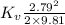`K_v(2.79^2)/(2*9.81)` = 4.898

or

`K_v=12.34`