Question

Need help with this maths question

Answer 1

XY = 18.6 mts

Angle of Depression = 61°

Explanation:

Let us start with finding out the Length of the XY

Y is directly on top of the center of the rectangular pool ABCD . Hence It must be on the top of the intersection point of the two diagonals. The length of XY will be half of Diagonal D .

Let us find Diagonal D by applying Pythagoras theorem in Triangle ABC

`D^2=AB^2 +BC^2`

`D^2=30^2+22^2`

`D^2= 900+484`

`D^2=1384`

`D=√(1384)`

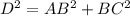
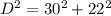
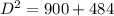
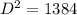
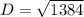
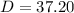`D=37.20` Approx

Hence Diagonal is 37.20 mts

Hence
`XY = (D)/(2)`

`XY=(37.20)/(2)`

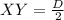
`XY=18.60` mts

Hence we have our first answer as 18.6 Mts

Part 2:

Please refer to the image attached with this answer.

The eye of the diver , the eye of the man standing on the pool , and the diagonal of the pool makes a right triangle A'O'Y'

Where

`O'Y' = 1.8-1.5+10 = 10.3`

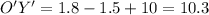
A'O' = half of the diagonal = 18.60 ( From first part of the problem)

Hence in ΔA'O'Y' , we have to determine ∠A'Y'O' or ∠x

Here applying trigonometric ratios

`\tan x = (opposite)/(adjacent)`

Opposite = A'O' = 18.60

Adjacent = Y'O' = 10.3

Hence

`\tan x = (18.60)/(10.30)`

`\tan x = 1.80`

`x=\tan^(-1)(1.8)`

`x=60.94`

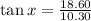
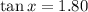
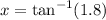
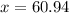
x≈61°

Hence Angle of depression for the diver towards the man standing at the pool edge would be 61°