Answer:
The correct option is D) 5:18.
Explanation:
Consider the provided information.
A husband and wife can complete a certain task in 1 and 2 hours respectively.
Then in 1 hr the work done by the husband and wife is:
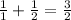
Thus, the work done is:

Rae and Herman, can complete the same task in 4 and 6 hours, respectively.
Then in 1 hr the work done by Rae and Herman is:
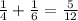
Thus, the work done is:
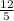
Now calculate the ratio as shown.
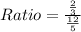
Ratio=5:18
Hence, the required ratio is 5:18.
Thus the correct option is D) 5:18.