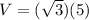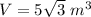Answer:
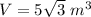
Explanation:
we know that
The volume of a trough is equal to
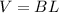
where
B is the area of equilateral triangle
L is the length of a trough
step 1
Find the area of equilateral triangle B
The area of a equilateral triangle applying the law of sines is equal to
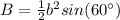
where

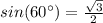
substitute
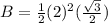
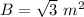
step 2
Find the volume of a trough
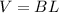
we have
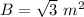
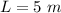
substitute