Question

Nunavet Ocean Cruises sold an issue of 12-year ​$1,000 par bonds to build new ships. The bonds pay​ 4.85% interest, semi-annually.​ Today's required rate of return is​ 9.7%. How much should these bonds sell for​ today? Round off to the nearest​ $1.

Answer 1

bond market value $660

Step-by-step explanation:

We need to calculate the present value of the maturity and the cuopon payment using the effective rate of 9.7%

First we do the annuity:

`C * (1-(1+r)^(-time) )/(rate) = PV\\`

C 24.25 (1,000 face value x 4.85 bond rate / 2 )

time 24.00 (12 year 2 payment a year)

rate 0.04850 (current rate divide by 2 to get it annually)

`24.25 * (1-(1+0.0485)^(-24) )/(0.0485) = PV\\`

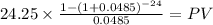
PV $339.55

Then present value of the maturity

`(Maturity)/((1 + rate)^(time) ) = PV`

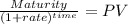
Maturity 1,000.00 the face value of the bond

time 24.00

rate 0.04850

`(1000)/((1 + 0.0485)^(24) ) = PV`

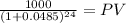
PV 320.89

Finally we add them together:

PV coupon payment $339.5545

PV maturity $320.8910

Total $660.4455

rounding to nearest dollar

bond market value $660