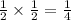Question

single die is rolled twice. Find the probability of rolling an oddodd number the first time and a number greater than 33 the second time.

Answer 1

`(1)/(4)`

Explanation:

A single die is rolled twice, we have to find the probability of rolling an odd number in first throw and a number greater than 3 in the second throw.

a) Rolling an Odd number in first throw

A die has total 6 possible outcomes, out of which 3 are odd numbers i.e. 1,3 and 5

So, total number of possible outcomes = 6

Total Favorable outcomes (Odd numbers) = 3

Probability is defined as the ratio of favorable outcomes to total number of outcomes. So,

The probability of rolling an odd number would be =
`(3)/(6) = (1)/(2)`

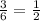
b) Rolling a number greater than 3 in second throw

Here again total possible outcomes = 6

Favorable outcomes (Numbers greater than 3 are 4, 5 and 6) = 3

So,

The probability of rolling a number greater than 3 =
`(3)/(6) = (1)/(2)`

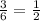
These two events(rolls) are independent of each other, so the overall probability of both events occurring would be the product of individual probabilities.

So,

Probability of rolling an odd number the first time and a number greater than 3 the second time =
`(1)/(2) * (1)/(2) = (1)/(4)`