Question

Suppose you want to make an open box out of a piece of card board by cutting small squares at the four corners and folding up the sides. If the piece of card board is a square whose sides are 1 m. long, how big a square should you cut from the corners to maximize the volume of the box?

Answer 1

The box of length
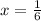`x=(1)/(6)` must be cut from each corner to maximize the volume.

Explanation:

It is given that the side of a square card board is 1 m.

Let small squares of length x are cut from the four corners. The dimensions of the box are

Length = (1-2x) m

Width = (1-2x) m

Height = x m

The volume of a cuboid is

`V=l* b* h`

where, l is length and b is breadth and h is height.

The volume of a rectangular box.

`V=(1-2x)(1-2x)x`

`V=(1-2x)(x-2x^2)`

`V=x-2x^2-2x(x-2x^2)`

`V=x-2x^2-2x^2+4x^3`

`V=4x^3-4x^2+x`

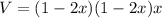
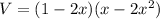
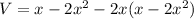
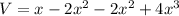
We need to maximize the volume. Differential above equation with respect to x.

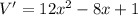`V'=12x^2-8x+1` .... (1)

Equate V'=0 to find the critical points.

`12x^2-8x+1=0`

`12x^2-6x-2x+1=0`

`6x(2x-1)-1(2x-1)=0`

`(6x-1)(2x-1)=0`

`x=(1)/(6),(1)/(2)`

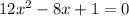
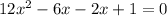
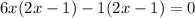
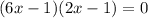
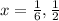
Differential equation (1) with respect to x.

`V''=24x-8`

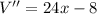
At
`x=(1)/(6)`

`V''=24((1)/(6))-8=-4<0`

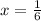
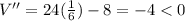
Volume is maximum at
`x=(1)/(6)`.

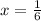At
`x=(1)/(2)`

`V''=24((1)/(2))-8=4>0`

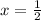
Volume is minimum at
`x=(1)/(6)`.

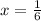Therefore the box of length
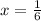`x=(1)/(6)` must be cut from each corner to maximize the volume.