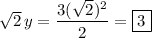An equilateral triangle with side length
 has area
has area
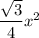 , so that
, so that
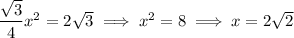
Then the triangle, and hence the square, has a perimeter of
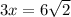 .
.
The perimeter of a square with side length
 is
is
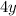 , so that
, so that

The length of the diagonal of any square is
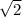 longer than the length of its side, so that this square's diagonal length is
longer than the length of its side, so that this square's diagonal length is