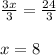Question

What is the justification for step 2 in the solution process?

10x − 25 − 3x = 4x − 1
Step 1: 7x − 25 = 4x − 1
Step 2: 7x = 4x + 24
A.
the multiplication property of equality
B.
the addition property of equality
C.
the division property of equality
D.
the subtraction property of equality

Answer 1

Answer: OPTION B.

Explanation:

Given the following equation:

`10x - 25 - 3x = 4x- 1`

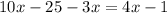
These are the steps to solve it:

Step 1

Add like terms on the left side of the equation:

`7x -25= 4x - 1`

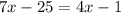
Step 2

Apply Addition property of equality, which states that:
`If\ a=b\ then\ a+c=b+c`

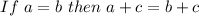
Then, we can add 25 to both sides of the equation:

`7x -25+(25)= 4x - 1+(25)\\\\7x=4x+24`

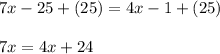
Step 3

Applying the Subtraction property of equality, we can subtract
`4x` from both sides:

`7x-4x=4x+24-(4x)\\\\3x=24`

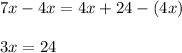
Step 4

Applying the Division property of equality, we can both sides of the equation by 3. Then:

`(3x)/(3)=(24)/(3)\\\\x=8`

Answer 2

B. the addition property of equality

Explanation:

we have:

Step 1: 7x − 25= 4x − 1

we apply the addition property of equality:

7x − 25+25= 4x − 1 +25

finally we have

Step 2: 7x = 4x + 24