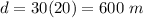Answer:
The length of the train is
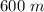
Explanation:
we know that
The speed is equal to the ratio of the distance by the time
Let
d -------> the distance in meters
t ------> the time in seconds
s -----> the speed in m/sec
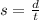
Remember that
1 km=1,000 m
1 h=3,600 sec
we have
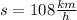
Convert to m/sec

Find the length of the train
we have
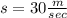

substitute in the formula and solve for d