Answer:
h = 13cm and b= 54cm.
Explanation:
We have that the area
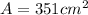 and the base is two centimeters longer than four times the height, that is
and the base is two centimeters longer than four times the height, that is
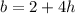
where b is the base and h the height. Now, the area is
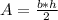
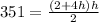
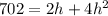
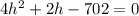 .
.
Now, we are going to use the general formula to solve quadratic ecuations:
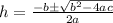
where a=4, b= 2 and c= -702.
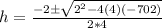
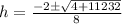
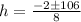
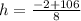 or
or

As we are searching for the lenght, we choose the positive result:
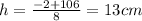
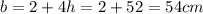 .
.