Question

Find the center and radius of the circle with equation (x + 3)^2 + (y – 6)^2 = 100.

Answer 1

center (-3,6)

radius(r) 10

Explanation:

The center-radius form (it is really called standard form) of a circle is:

`(x-h)^2+(y-k)^2=r^2`

where
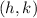`(h,k)` is the center and
`r` is the radius.

Compare the following:

`(x-h)^2+(y-k)^2=r^2`

`(x+3)^2+(y-6)^2=100`

You should see the following:

`-h=3`

`-k=-6`

`r^2=100`.

`-h=3 \text{ implies }h=-3`.

`-k=-6 \text{ implies } k=6`.

`r^2=100 \text{ implies } r=√(100)=10`.

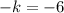
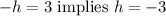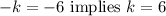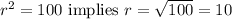So the center is (-3,6) and the radius is 10.