Question

use compound angle formulae to find sin 15 and cos 15 in form of [ surd a (surd b + c) ] . Hence, find tan 15 in the simplest surd form​

Answer 1

see explanation

Explanation:

Using the difference formulae for sine and cosine

sin(x - y) = sinxcosy - cosxsiny

cos(x - y) = cosxcosy + sinxsiny

sin15° = sin(45 - 30)°

sin(45 - 30)°

= sin45°cos30° - cos45°sin30°

=
`(√(2) )/(2)` ×
`(√(3) )/(2)` -
`(√(2) )/(2)` ×
`(1)/(2)`

=
`(√(6) )/(4)` -
`(√(2) )/(4)`

=
`(√(6)-√(2)  )/(4)`

----------------------------------------------------------------------------------

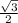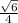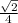
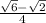
cos15° = cos(45 - 30)°

cos(45 - 30)°

= cos45°cos30° + sin45°sin30°

=
`(√(2) )/(2)` ×
`(√(3) )/(2)` +
`(√(2) )/(2)` ×
`(1)/(2)`

=
`(√(6) )/(4)` +
`(√(2) )/(4)`

=
`(√(6)+√(2)  )/(4)`

-------------------------------------------------------------------------------------

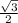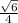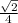
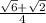
tan15° =
`(sin15)/(cos15)`

=
`(√(6)-√(2)  )/(4)` ×
`(4)/(√(6)+√(2)  )`

=
`(√(6)-√(2)  )/(√(6)+√(2)  )`

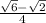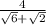
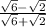
Rationalise the denominator by multiplying numerator/ denominator by the conjugate of the denominator, that is (
`√(6)` -
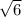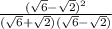
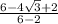
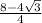
`√(2)` )

=
`((√(6)-√(2))^2  )/((√(6)+√(2))(√(6)-√(2))    )`

=
`(6-4√(3)+2 )/(6-2)`

=
`(8-4√(3) )/(4)`

= 2 -
`√(3)`