Answer: 276 days
Step-by-step explanation:
This problem can be solved using the Radioactive Half Life Formula:
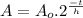 (1)
(1)
Where:
 is the final amount of the material
is the final amount of the material
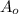 is the initial amount of the material
is the initial amount of the material
 is the time elapsed
is the time elapsed
 is the half life of polonium-210
is the half life of polonium-210
Knowing this, let's substitute the values and find
 from (1):
from (1):
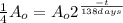 (2)
(2)
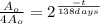 (3)
(3)
 (4)
(4)
Applying natural logarithm in both sides:
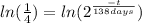 (5)
(5)
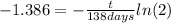 (6)
(6)
Clearing
 :
:
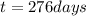 (7)
(7)