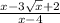Question

Can you help me with 8b and 8c and explain it please

Answer 1

8b) 2x+
`√(3)`x

8c)
`(x-3√(x) +2 )/(x-4)`

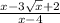
Explanation:

8b)

Multiply the top and bottom by 2+
`√(3)` to rationalize this fraction

`(x)/(2-√(3) ) * (2+√(3) )/(2+√(3) )`

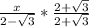
Simplify the product

`(x(2+√(3)) )/(1)`

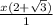
Distribute x through the parenthesis

2x+
`√(3)`x

8c)

Multiply the top and bottom by
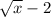`√(x)-2` to rationalize this fraction

`((√(x)-1)*(√(x)-2) )/((√(x)+2)*(√(x)-2) )`

Simplify the product

`(x-2√(x)-√(x)+2  )/(x-4)`

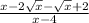
Collect like terms

`(x-3√(x) +2 )/(x-4)`